Calculation Example – Cantilever Beam, Temperature change

Contents [show]
The beam is fixed at end B and free at end A. It is loaded at end A with the concentrated force 2F. At the same time there is a temperature change ΔT. Calculate the length change Δx. (Modulus of elasticity E, Cross section area AS , Temperature coefficient a.)
Solution There are two causes for the length change. The first cause for the length change is force 2F. The area of the cross section of the beam is AS so the stress is ?=2F / AS . The change in length is ?x1 and the deformation of the beam is ?1= ?x1/x. The Hook law gives : ;
Selected Topics
Want to read more like this?

Temperature Change
Sep, 30, 2019 | EducationThe beam is fixed at end B and free at end A. It is loaded at end A with the concentrated force 4F....

FBEAM 2011
Nov, 05, 2013 | Software
Calculation Example: Calculate the Change in Length of a Rod loaded in extension
Jan, 12, 2016 | EducationThe rod of the picture is loaded with the force P2 which is uniformly distributed on Section D where...

Calculation Example – Cantilever Beam
Jul, 25, 2016 | EducationFind the member diagrams for the triangular loading. SOLUTION We calculate the reactions at the...

Calculation Example – Cantilever Beam with uniform loading.
Oct, 04, 2017 | EducationCalculate the internal forces for the cantilever beam with the uniform loading q=10KN/m. &nbs...
Beams
Sep, 14, 2023 | EducationBeam, in structural engineering, is a horizontal structural element that is designed to carry and...

Calculation Example – Calculate shear stress for temperature load.
Oct, 04, 2017 | EducationA copper bar with a rectangular cross section is held without stress between rigid supports. The tem...

Calculation Example - Calculate the member diagrams for the beam
Feb, 16, 2016 | EducationCalculate the member diagrams for Axial Force N, Shear Force Q and bending Moment M for the followin...

Calculation Example - Calculate the member diagrams.
Jan, 25, 2017 | EducationCalculate the member diagrams for the point load P for the pinned beam at two ends. Solut...
Trending

Diaphragms
Structural stability
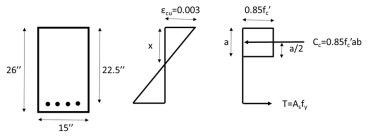
Nominal flexural strength of a reinforced concrete beam

Calculate the Maximum Shear Stress
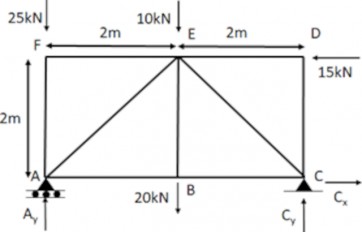
Truss deflection using the unit load method

Overhanging beam: shear force and bending moment calculation

Calculation Example – Beam with inner hinge (Part A). Find the Reactions

